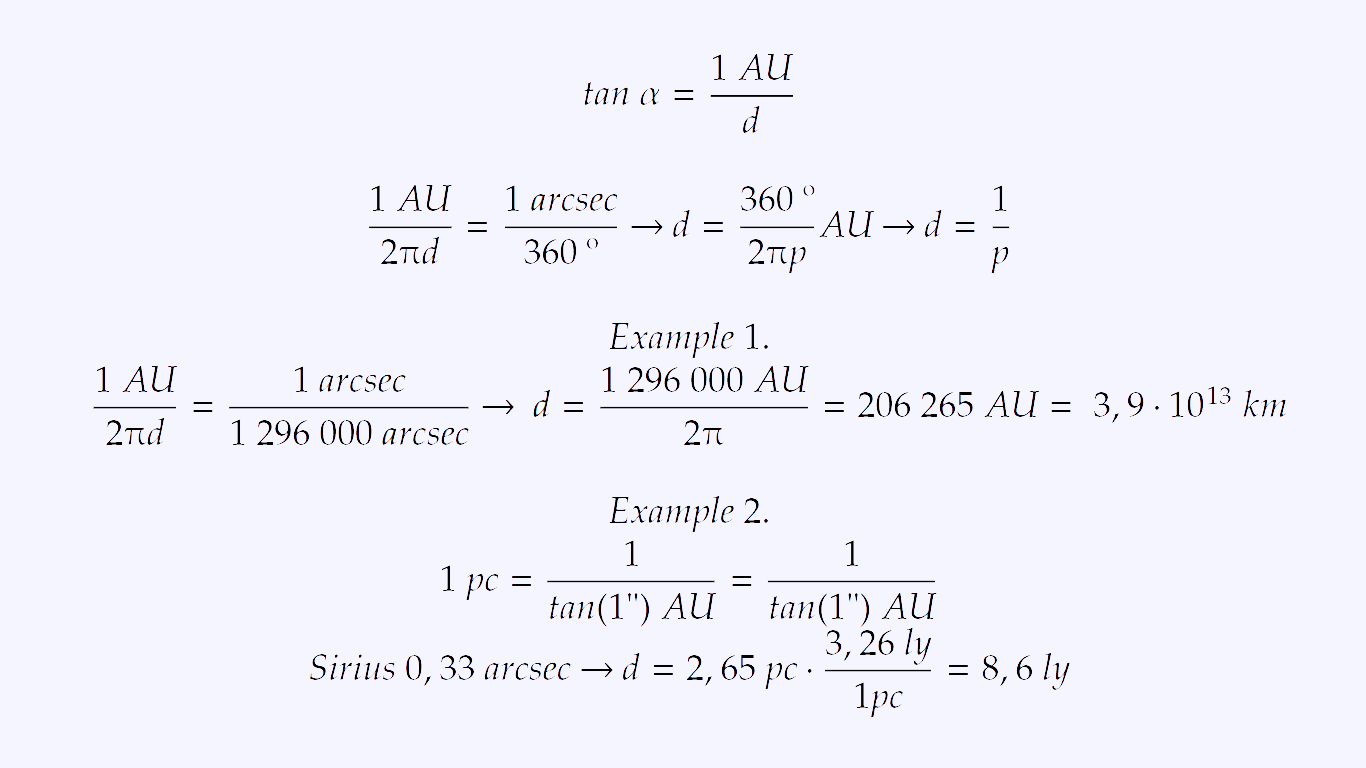
Parallax

Dual perspective allows to get a general sense of how far away objects are. Let's see how astronomers take advantage of this idea.

An astronomer is trying to measure the distance to a star from Earth. Seen from opposite sides of Earth's orbit (for example, on January and July), this nearby star shifts its position when compared to a pattern of more distant stars. This apparent change in direction of the remote object due to a change in vantage point of the observer is called parallax (triangulation).

Illustration of Parallax. The Sun is drawn as a yellow disk in the left hand portion of the diagram and is labeled “Sun.” A blue circle surrounds the Sun and is labeled “Earth’s orbit.” The Earth is shown at two positions on the blue circle. Position “A” at the bottom of the circle and “B” at the top. Above and to the right of the center, a nearby star is drawn as an unlabeled red dot. In the upper right is an unlabeled group of five stars that are more distant than the red star. A white line is drawn from position A through the red dot to the uppermost stars in the group. A white line is drawn from position B through the red dot to the middle star in the group. A dashed line is drawn from the Sun to the red dot. The parallax angle, “p,” is drawn between the dashed line and line B. To illustrate the effect of parallax, two insets are included near points A and B. The inset at point B is labeled “Sky as seen from B,” and shows the red dot near the middle star of the group of five stars that are illustrated in the upper right side of the figure. The inset at point A is labeled “Sky as seen from A,” and shows the red dot near the uppermost stars of the group of five stars that are illustrated in the upper right side of the figure.

Through this method we can obtain the distance of a star from Earth.

IMPORTANT UNITS

1 AU	Astronomical Unit	1,5 ∙ 10^8 km
1 pc	Parsec	3,6 ly
1 ly	Light year	9.5 trillion km

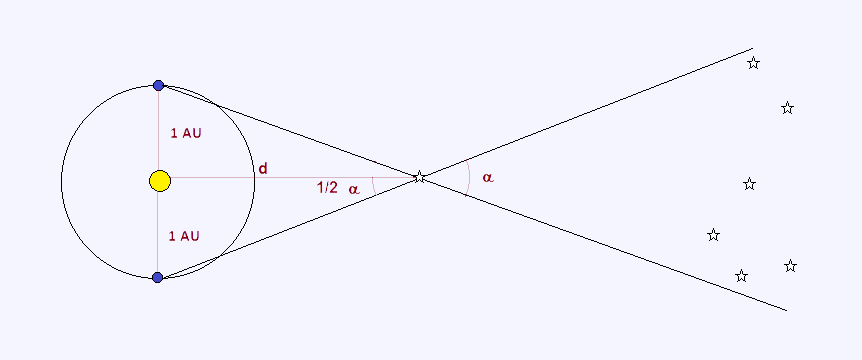
Using trigonometry on the previous graph we can state that: